IB Physics HL Topic 12

A changing magnetic field induces an emf — current within a conductor. Such a current is called an induced current. Motion or change is required to induce an emf. If does not matter whether the magnet or the coil moves.

See image.

1. *Magnetic Flux (Φ) - product of the magnetic field strength and a cross-sectional area and the cosine of the angle between the magnetic field and the normal to the area (Φ = B A cosθ) 2. *Magnetic Flux Linkage – product of the magnetic flux through a single coil and the total number of coils (flux linkage = N Φ)

See image. A time changing magnetic flux will induce an emf in a circuit or coil (conductor).

1. *Faraday’s Law - The emf induced by a time changing magnetic field is proportional to the rate of change of the flux linkage. (ε α N ΔΦ/Δt) 2. *Lenz’s Law - The direction of an induced emf is such that it produces a magnetic field whose direction opposes the change in magnetic field that produced it. (NOTE: This is the negative sign added to Faraday’s law. ε= - N ΔΦ/Δt)

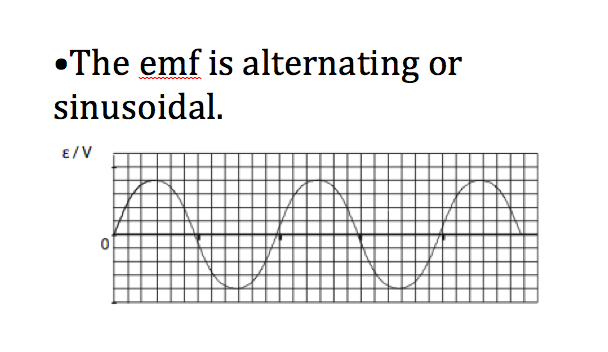See image. Students should understand, without any derivation, that the induced emf is sinusoidal if the rotation is at constant speed.

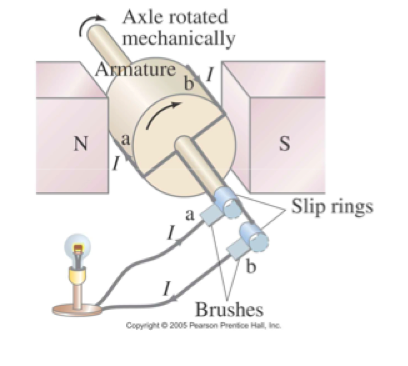A generator consists of many loops of wire wound on an armature that can rotate in a magnetic field. The axle is turned by some mechanical means and an emf is induced in the coil.

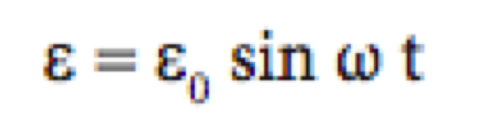Students will be expected to compare the output from generators operating at different frequencies by sketching appropriate graphs.

Students should know that the rms value of an alternating current (or voltage) is that value of the direct current (or voltage) that dissipates power in a resistor at the same rate. The rms value is also known as the rating.

See image.

See image.

Power loss in transmission line:
Heat loss due to current Resistance of the metal used Dielectric losses Self-inductance Power loss in real transformers:
Eddy currents Resistance of the wire in the winding Hysteresis Physical Vibration Dielectric losses Electromagnetic radiation Flux leakage

Students should be aware that, for economic reasons, there is no ideal value of voltage for electrical transmission.
The voltage is step-up from the power plant to have a higher power output due to power dissipating. The voltage is then step-down when the power has reached its consumer source (where the power is being consumed). There is no ideal valve of step-up voltage due to economic reason. From the power plant, a step-up transformer is used to increase voltage (so that current is decreased) from 11,000 V to 250,000 V. from the transmission line to city storage, a step-down transformer reduces voltage from 250,000 to 4500 V. then in front of a house, a step-down transformer reduces that to 200-240V.

A human body is a conducting medium, so when it is moving in an alternating magnetic field at extra-low-frequency, then electric field is induced, hence inducing current in human body.

Students should be aware that current experimental evidence suggests that low-frequency fields do not harm genetic material. Students should appreciate that the risks attached to the inducing of current in the body are not fully understood. These risks are likely to be dependent on current (density), frequency and length of exposure.
Current experimental evidence suggests that low-frequency fields don’t harm genetic material. There has been evidence that this may lead to infant cancer and infant leukemia.